mildly Hausdorff
Last-modified: 2013-08-24 (土) 00:22:59
Definition 
Property 
- For a topological space (X,τ), the following conditions are equivalent;
- X is mildly Hausdorff.
- For any open set U and any point x ∈ U, there is a δ-generalized closed set? F such that x ∈ F ⊆ U.
- Every open set is η-open.
- Every closed set is the intersection of δ-open sets.
- Every mildly Hausdorff strongly S-closed? space is locally indiscrete? and hence extremally disconnected? and (semi-)compact?.
- Let
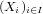 be a family of Topological spaces. For the topological sum
be a family of Topological spaces. For the topological sum
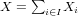 the following conditions are equivalent:
the following conditions are equivalent:
- X is mildly Hausdorff.
- Each X_i is mildly Hausdorff.
- Let (X_i, τ_i) be mildly Hausdorff for each i ∈ I. If
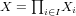 and τ is the product topology on X, then X is mildly Hausdorff.
and τ is the product topology on X, then X is mildly Hausdorff.
- Every (weakly) mildly Hausdorff space is I-compact?.
Reference 
- Dontchev, J.; Popvassilev, S.; Stavrova, D., On the η-expansion topology for the co-semi-regularization and mildly Hausdorff spaces., (English summary), Acta Math. Hungar. 80 (1998), no. 1-2, 9-19.


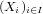 be a family of Topological spaces. For the topological sum
be a family of Topological spaces. For the topological sum
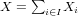 the following conditions are equivalent:
the following conditions are equivalent:
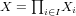 and τ is the product topology on X, then X is mildly Hausdorff.
and τ is the product topology on X, then X is mildly Hausdorff.
 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ