p-regular
Last-modified: 2012-10-04 (木) 14:33:34
Definition 
- A topological space (X,τ) is said to be p-regular if for each closed set F of X and each point
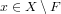 , there exist disjoint pre-open? sets U and V such that
, there exist disjoint pre-open? sets U and V such that
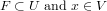 .
.
Property 
- For a topological space (X,τ) the following are equivalent:
- X is p-regular.
- For each x in X and each open set U of X containing x, there exists pre-open? set V such that
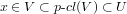 .
.
- For each closed set F of X,
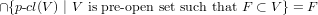
- For each subset A of X and each open set U of X such that
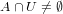 , there exists pre-openset? V such taht
, there exists pre-openset? V such taht
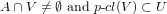 .
.
- For each nonempty subset A of X and each closed set F of X such tant
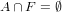 , there exist pre-open? sets V and W such that
, there exist pre-open? sets V and W such that
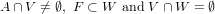 .
.
- The product space of p-regular spaces is p-regular.
- If X is a p-regular space and X' is an α-set of X, then the subspace X' is p-regular.
Reference 
- El-Deeb, N.; Hasanein, I. A.; Mashhour, A. S.; Noiri, T. , On p-regular spaces., Bull. Math. Soc. Sci. Math. R. S. Roumanie (N.S.) 27(75) (1983), no. 4, 311–315.

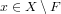 , there exist disjoint pre-open? sets U and V such that
, there exist disjoint pre-open? sets U and V such that
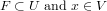 .
.
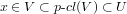 .
.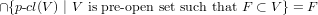
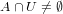 , there exists pre-openset? V such taht
, there exists pre-openset? V such taht
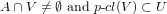 .
.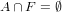 , there exist pre-open? sets V and W such that
, there exist pre-open? sets V and W such that
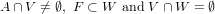 .
.
 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ