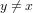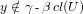γ-βT_2
Last-modified: 2011-08-20 (土) 12:51:27
Definition 
- A topological space (X,τ) with an operation γ on τ is called γ-βT_2 if and only if for each pair of distinct points x, y in X, there exists two disjoint γ-β-open sets U and V containing x and y respectively.
Property 
- The following are equivalent for a topological space (X,τ) with an operation γ on τ :
- X is γ-βT_2.
- Let x ∈ X. For each
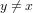 , there exists a γ-β-open set U containing x such that
, there exists a γ-β-open set U containing x such that
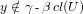 .
.
- For each x ∈ X, ∩{γ-βcl(U) : x ∈ U ∈ γ-βO(X)} = {x}.
- γ-βT_2 ⇒ γ-βT_1.
Reference 
- Basu, C. K.; Afsan, B. M. Uzzal; Ghosh, M. K., A class of functions and separation axioms with respect to an operation. (English summary), Hacet. J. Math. Stat. 38 (2009), no. 2, 103–118.



 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ