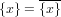T_{FF}
Last-modified: 2013-04-19 (金) 13:20:03
Definition 
- A topological space (X,τ) is said to be T_{FF} if for any two disjoint, finite sets F_1 and F_2 either F_1 is weakly separated from F_2 of F_2 is weakly separated form F_1
Property 
- T_{FF} implies T_Y.
- A topological space (X,τ) is a T_{FF} space if and only if either
-
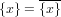 for all but at most one x ∈ X, or
for all but at most one x ∈ X, or
- {x} = ker(x) for all but at most one x ∈ X. [2]
Reference 
- Aull, Charles E.; Thron, W.J.,Separation axioms between T0 and T1., (English) [J] Nederl. Akad. Wet., Proc., Ser. A 65, 26-37 (1962).
- Johnston, B.; McCartan, S. D., Minimal T_F-spaces and minimal T_FF-spaces. Proc. Roy. Irish Acad. Sect. A 80 (1980), no. 1, 93–96.



 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ