Definition 
- A Hausdorff space (X, τ) is said to be a strongly Hausdorff space if for each infinite subset A ⊆ X, there is a sequence { U_n : n∈N } of pairwise disjoint open sets such that
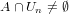
Property 
- strongly Hausdorff ⇔ T_0 + strongly R_1. [2]
Reference 
- Porter J. R., Strongly Hausdorff spaces. Acta Math. Acad. Sci. Hungar. 25 (1974), 245–248.
- Dorsett Charles, Strongly R1 spaces., Kyungpook Math. J. 21 (1981), no. 2, 155–161.
 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ