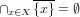*Definition [#tc9b9895]
-Let (X,τ)　be a topological space. X is said to be weakly R_0 , briefly w-R_0 , if &ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b%5c%5b%20%5ccap_%7bx%5cin%20X%7d%5coverline%7b%5c%7bx%5c%7d%7d=%5cemptyset%20%5c%5d%7d%25.png);.

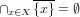*Property [#ocebf5ec]
-A topological space (X,τ) is weakly-R_0 iff for each x in X, ker(x) ≠ X. 
-If a space X is  w-R_0, then for every topological space Y, the product space X×Y is also w-R_0.
-If a product X×Y is w-R_0, the at least one of the factor is w-R_0.
-weakly R_0 ⇒ [[weakly semi-R_0]]. [2]

*Reference [#e328f153]
+Di Maio, Giuseppe, ''A separation axiom weaker than R0.'', (English) [J] Indian J. Pure Appl. Math. 16, 373-375 (1985).
+Arya, S.P.; Nour, T.M., ''Weakly semi-R_0 spaces.'', (English) [J] Indian J. Pure Appl. Math. 21, No.12, 1083-1085 (1990).