Top > locally compact

locally compact

Last-modified: 2010-12-04 (土) 16:27:49

Definition

A topological space X is called locally compact if every point of X has a compact neighbourhood.

Property

Every locally compact Hausdorff space is Tikhonov?.
Every locally compact, paracompact Hausdorff space is strongly paracompact.
For every compact subspace A of a locally compact space X and every open set V that contains A there exists an open set U such that 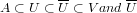is compact.
If X is locally compact space, then every subspace of X that can be represented in the form F∩V, where F is closed in X and V is open in X, also is locally compact.
Every locally compact subspace M of a Hausdorff space X is an open subset of the closure of the set M in the space X, i.e., it can be represented in the form F∩V, where F is closed in X and V is open in X.
The sum is locally compact if and only if all spaces X_s are locally compact.
The Cartesian product 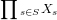, where , is locally compact if and only if all spaces X_s are locally compact and there exists a finite set such that X_s is compact for .
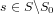If there exists on open mapping f:X→Y of a locally compact space X onto a Hausdorff space Y, then Y is a locally compact space.
For every locally compact space X and any quotient mapping g:Y→Z, the Cartesian product f=Id_X×g;X×Y→X×Z is a quotient mapping.
If X is locally compact, then for every topological space Y the compact-open topology on Y^X is acceptable.
Every locally compact paracompact space X can be represented as the union of a family of disjoint closed-and-open subspaces of X each of which has the Lindeloef property.
Every non-empty hereditarily disconnected locally compact space is zero-dimensional.
Hereditary disconnectedness, zero-dimensionality and strong zero-dimensionality are equivalent in the realm of non-empty locally compact paracompact spaces.
For every non-emty locally compact paracompact space X the conditions ind X=0, Ind X=0 and dim X=0 are equivalent to hereditary disconnectedness of X.
a coarsest uniformity? on a Tykhonov? space X exists if and only if the space X is locally compact.
Every locally compact preregular space is completely regular.
Every locally compact Hausdorff space is a Baire space.
A subspace X of a locally compact Hausdorff space Y is locally compact if and only if X can be written as the set-theoretic difference of two closed subsets of Y.