p-compact

Definition

p denotes a free ultrafilter on ω, the set of natural numbers.
Let X be a topological space, (S_n) a sequence of nonempty subsets in X. A point x in X is called a p-limit point of (S_n) if for all neighborhood V of x, .
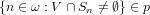If (x_n) is a sequence of points in X, "a p-limit point of (x_n)" means a p-limit point of a sequence of singleton ({x_n}).
X is said to be p-compact if every sequence (x_n) has a p-limit point.

Remark

• If X is p-compact for all p, X is called ultracompact.
• For the term in bitopology, see p-compact in bitopology.

Reference

• A. R. Bernstein, A new kind of compactness for topological spaces, Fund.Math. 66 (1970), 185-193.