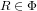Dugundji compact

Definition

• a compact space X is Dugundji if it has one of the following equivalent properties:
  1. if Z is a zero-dimensional compact space and A is a closed subset of Z, then
     every continuous map A → X extends to a continuous map Z → X;
  2. there exists a family Φ of closed equivalence relations on X such that:
     1. for every the quotient space X/R is metrizable and the quotient map X → X/R is open;
     2. ∩Φ = the diagonal of X^2 (in other words, the family Φ separates points of X);
     3. Φ is closed under countable intersections.

Property

• For every topological group X every compact G_δ-subset of X is Dugundji.
• For every Mul’tsetv space? X every compact G_δ-subspace of X is Dugundji.

Reference

• Reznichenko, E. A.(RS-MOSCM-GT) and Uspenskij, V. V.(RS-MOSCM-GT), Pseudocompact Malʹtsev spaces. (English summary) ,Special issue on topological groups. ,Topology Appl. 86 (1998), no. 1, 83--104.