co-compact

Definition 1

• A topological space X is co-compact if any co-open cover of X has a finite subcover.

Definition 2

A topological space X is said to be co-compact if there is a collection D of a closed subsets which satisfies the following:

1. any subcollection of D with fip has nonempty intersection;
2. if U is an open subset of X and if x is a point in U, then there is some V in D with .

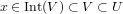Reference

Definition 1

• Abd El-Monsef, M. E.(ET-TANT) and Kozae, A. M.(ET-TANT),Remarks on $s$-closed spaces (Arabic summary), Qatar Univ. Sci. Bull. 6 (1986), 11--21.

Definition 2

• H. Bennett and D. J. Lutzer, Strong completeness properties in topology, Questions and Answers in General Topology, 27(2009), 107-124.
• http://www.math.wm.edu/~lutzer/drafts/BigBushes.pdf (preprint)