F-compact
Last-modified: 2011-10-25 (火) 19:17:40
Definition 
- A topological space X is called F-compact if every open cover of X contains of an F-finite subcover (F-finiteness stands for any reasonable definition of finiteness).
Finiteness 
- A set S is said to be
- I-finite if every nonvoid family of subsets of S has an
 -maximal element,
-maximal element,
- I_a-finite if it is not the union of two disjoint sets neither of which is finite according to definition I,
- II-finite if every non-void
 -monotone familiy has a
-monotone familiy has a
 -maximal element,
-maximal element,
- III-fnite if the power set of S is irreflexive (i.e., there is no one-to-one mapping of P(S) onto a proper subset of P(S)),
- IV-finite if it is irreflexive,
- V-finite if |S|=0 or 2. |S|>|S|,
- VI-finite if |S|=0 or |S|=1 or |S|^2>|S|,
- VII-finite if S is Ⅰ-finite or S is not well-orderable.
- Let F very over I,I_a,II,III,IV,V,VI,VII. We say that a set S is F"-finite if P(S) is F-finite.
- In ZF (or ZFU) the following implications are provable;
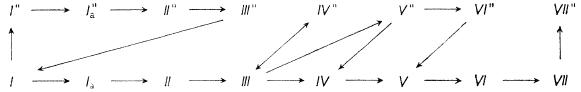
Remark 
Reference 
- Spisiak, Ladislav and Vojtas, Peter, Dependences between definitions of finiteness., Czech. Math. J. 38(113), No.3, 389-397 (1988).


 -maximal element,
-maximal element, -monotone familiy has a
-monotone familiy has a
 -maximal element,
-maximal element,

 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ