c-realcompact

Last-modified: 2010-12-30 (木) 16:59:06

Definition

Let X be a Tychonoff space. 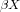imgtex.fcgi?%5bres=100%5d%7b$%5cbeta%20X$%7d%25.png denotes the Stone-Cech compactification of X. X is called c-realcompact if for every 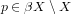imgtex.fcgi?%5bres=100%5d%7b$p%5cin%5cbeta%20X%5csetminus%20X$%7d%25.png , there exists a normal lower semicontinuous function f on 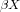imgtex.fcgi?%5bres=100%5d%7b$%5cbeta%20X$%7d%25.png such that f(p) = 0 and f is positive on X.

Property

  • A Tychonoff space X is c-realcompact iff for every 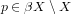imgtex.fcgi?%5bres=100%5d%7b$p%5cin%5cbeta%20X%5csetminus%20X$%7d%25.png , there is a sequence of regular closed subsets of 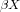imgtex.fcgi?%5bres=100%5d%7b$%5cbeta%20X$%7d%25.png which satisfies 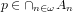imgtex.fcgi?%5bres=100%5d%7b$p%5cin%20%5ccap_%7bn%5cin%20%5comega%7dA_n$%7d%25.png and imgtex.fcgi?%5bres=100%5d%7b$%5ccap_%7bn%5cin%20%5comega%7d%28A_n%5ccap%20X%29=%5cemptyset$%7d%25.png .

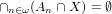Reference

  • Nancy Dykes, Generalizations of Realcompact Spaces, Pacific Journal of Mathematics Vol. 33, No. 3, 1970.
  • Mary Anne Swardson and Paul J. Szeptycki, When X^* is a P' space, Canad. Math. Bull. Vol. 39 (4), 1996 pp. 476-485.