Definition
A space X is called countably almost preorthocompact provided that, if C is a countable open cover of X, there is a reflexive relation V on X so that, for each z in X, V(z) is open and whenever y is in
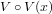and x is in V(z), {x, y} is a subset of some member of C.
Reference
- Hans-Peter Kunzi and Peter Fletcher, Some Questions Related to Almost 2-Fully Normal Spaces, Rocky Mountain J. Math. Vol.15 (Nov. 1985).