mildly compact

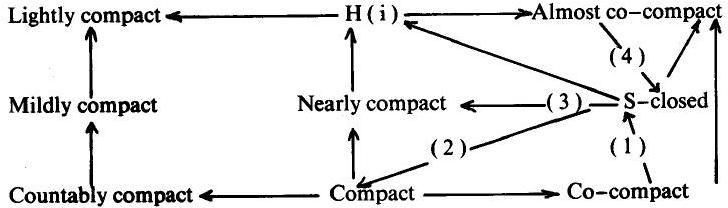
Definition 1

A topological space X is mildly compact if any countable open cover of X has a finite subfamily the interiors of the closures of whose members cover X.

Property

Reference

Abd El-Monsef, M. E.(ET-TANT) and Kozae, A. M.(ET-TANT),Remarks on $s$-closed spaces (Arabic summary), Qatar Univ. Sci. Bull. 6 (1986), 11--21.

Definition 2

A topological space X is called mildly compact if every clopen cover has a finite subcover.

Reference

R. Staum, The algebra of bounded continuous functions into a nonarchimedean field, Pacific J. Math., 50 (1974), 169-185.