*Definition [#j96b2e47]
A T_1 space X is called superparacompact if every open cover has an open [[finite component refinement>finite component cover]].

*Property [#mf09e4d0]
Let X be a completely regular space and let 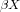&ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$%5cbeta%20X$%7d%25.png); be its Stone-Cech compactification. X is superparacompact iff for every [[compact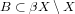]] &ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$B%5csubset%5cbeta%20X%5csetminus%20X$%7d%25.png);, there exists a [[finite component cover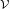]] &ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$%5cmathcal%7bV%7d$%7d%25.png); of the space X such that 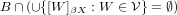&ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$B%5ccap%20%28%5ccup%5c%7b%20%5bW%5d_%7b%5cbeta%20X%7d:W%5cin%20%5cmathcal%7bV%7d%5c%7d%20=%5cemptyset%29$%7d%25.png);, where 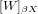&ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$%5bW%5d_%7b%5cbeta%20X%7d$%7d%25.png); denotes the closure of W in &ref(http://www.eaflux.com/imgtex/imgtex.fcgi?%5bres=100%5d%7b$%5cbeta%20X$%7d%25.png);.
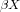*Reference [#j187f758]
-D. Buhagiar and T. Miwa, ''On superparacompact and Lindeloef GO-spaces'', Houston J. Math. Vol.24, No.3, 1998.
-D. Buhagiar, T. Miwa, and B. A. Pasynkov, Superparacompact type properties, Yokohama Math. J. Vol.46, pp.71-86, 1998.