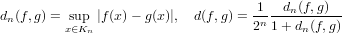Definition
A topological space is said to be hemicompact if there is a sequence of compact subsets (called admissible sequence) such that every compact subset is contained in some member of the sequence.
Property
- A hemicompact space is the union of the admissible sequence since every singleton in the space is a compact set.
- Every first countable hemicompact space is locally compact.
- If X is hemicompact, then the space C(X) of all continuous functions from X to the real is metrizable. The metric is given by where K_n are the admissible sequence.
Reference
Willard, Stephen, General Topology, Dover (2004).