Definition 
- A topological space is called stably compact if it is;
- T_0
- compact;
- locally compact;
- coherent, that is, intersections of compact saturated sets are compact;
- well-filtered, that is, if the intersection of a filter base
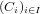 of compact saturated sets is contained in an open set, then so is some C_i already.
of compact saturated sets is contained in an open set, then so is some C_i already.
Reference 
- Achim Jung and M. Andrew Moshier, On the bitopological nature of Stone duality (2006)
 新規
新規 編集
編集 添付
添付 一覧
一覧 最終更新
最終更新 差分
差分 バックアップ
バックアップ 凍結
凍結 複製
複製 名前変更
名前変更 ヘルプ
ヘルプ